WORLD FOR CIVIL

Experiment 5

Stress and Strain

An Introduction to Stress and Strain

Objective: Students will design and make a concrete cylinder and beam that can withstand the greatest applied load.

Scientific Principles:

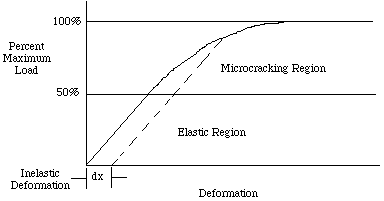
Solids have rigidity because the bonding forces between the atoms resist changes. When an external force is applied to a solid, the interatomic forces allow for deformations to occur. When the distorting external force is removed, the forces between the atoms bring them back to their original positions and the solid, as a whole, back to its original shape. This property is called elasticity. All solid materials are elastic to some extent, even concrete. The amount of elastic deformation that can occur in a material is dependent on the type of stress applied.

Two common types of stress are compressive and tensile. Compressive forces exist when you push together a material. Walking on concrete is one such example. Tensile stresses describe a material when it is pulled upon. The cable that supports the load of a crane is a good example of tensile stresses.

While in elastic regions, compressive and tensile stresses do no damage to the material. A material will return to its original dimensions once the stress has been removed if it is within this region. If the stress becomes too great in concrete, microscopic cracks begin to form inside the concrete. Once cracks begin to form, the concrete will not return exactly to its original dimensions and thus behaves inelastically. These changes are very small and not easy to measure without expensive equipment. As the load increases, these microscopic cracks come together so as to form one large macroscopic crack, and the concrete fractures or fails. Concrete has very good compressive properties but very poor tensile properties unless steel reinforcements are added.

Time: 90 min. (1 class period for making the beams & cylinders and 1 period for testing)

Materials and Supplies:

The following materials are needed for each group of 2-3 students:

• cement
• various kinds of aggregate
• mixing containers
• stirring utensils
• forms for making beams and cylinders (enough for each student to work individually)

LOW-TECH COMPRESSION TESTER

• two 8' long 2x4's
• one 2' long 2x4
• large gate hinge
• sheet metal -cut into two 4 "squares
• 2 copper pipe caps to fit over concrete cylinders
• 2 rubber stoppers or washers to fit inside pipe caps

MEDIUM TECH COMPRESSION TESTER

• 2 C-clamps ---6" span or larger
• 2 copper pipe caps to fit over concrete cylinders
• PVC pipe slightly longer than concrete cylinders and slightly larger in diameter
• 2 rubber stoppers or washers to fit inside pipe caps

HIGH TECH COMPRESSION TESTER

• hydraulic jack with pressure gauge
• 2 copper pipe caps to fit over concrete cylinders
• 2 rubber stoppers or washers to fit inside copper caps

Design Competition Parameters:

1. All cylinders and beams will be made using uniform molds which will be provided.
2. The concrete used to make each cylinder and beam must be made using the raw materials provided.
3. Suggested recipe for concrete is 3 parts aggregate, 2 parts cement, and 1 part water. Any combination of aggregate or none at all may be used. The exact amounts of each component to be used is up to you!
4. The beams must span at least 30 centimeters but may be longer.

Beam Testing Procedure:

1. Support the beam between two tables of the same height.
2. Twist the ends of a wire together so that it forms a circle around a bucket handle. Put this loop around the middle of the beam.
3. Add weight to the bucket in uniform increments. Wait 30 seconds between each addition of weight.
4. Continue to add weight until the beam breaks.

Cylinder Testing Procedure:

1. Place the cylinder in the testing apparatus.
2. Apply force until the concrete is smashed.

Video Clip Making of the Cylinder

Video Clip Smashing the Cylinder

Questions and Analysis:

1. If a horizontal concrete beam is supported at one end and a downwards force is applied to the other end, where would the microscopic cracks tend to be formed? The top layer, the bottom layer, or the middle layer?

2. If a concrete beam is supported but not held at both ends and a load is applied in the middle, which layer would be under compression? The top layer, the bottom layer, or the middle layer?

3. In the construction of a bridge, would concrete be better suited for the columns supporting the bridge or for the bridge decking? Explain your answer.

4. Write the formula needed to calculate the
   a. tensile strength of a beam
   b. compression strength of a cylinder

5. Calculate the strength each of your structures exhibited in the competitions.

6. a. Write the equation to calculate the force exerted on a cylinder.
   b. Calculate the force that was exerted on your cylinder.

7. What changes would you make to improve your beam design?

8. What changes would you make to improve your cylinder design?

Notes for Teacher:

We used a 24" truss spacer as our beam forms. These can be found at a hardware store. Our truss spacers cost 69 cents each and are reusable. A toothpaste box reinforced with tape can be used as a form. The plastic cases that toothbrushes come in could also be used. However, obtaining a classroom set of these might prove to be a challenge. The beam testing could also be set up as a cantilever style with only one end of the beam fixed and the load applied to the free end.

Free weights can be added to the bucket until breakage occurs. If free weights are not available, water can be used. Be careful of water spillage.

Cylindrical forms can be plastic or paper tubes, pipe insulation, or any other small diameter tubing. The concrete needs to be easily removable from the forms. Pipe insulation from a hardware store works well. This foam material is easy to work with, reusable, and inexpensive. The bottom of the forms can be taped, corked, or set on glass plates. The goal is to achieve smooth top and bottom surfaces which are necessary when performing the compression test. If the surface is not smooth, the force will be applied unequally. We recommend the cylinders be no more than 1/2" in diameter. Cylinders with a larger diameter will require a greater force to fail. A good rule of thumb is the height of the cylinder should be at least twice the diameter of the cylinder. However, if the cylinder is too tall it will tend to buckle.

The aggregate selection could be limited to just sand or could include gravel. The strength of the specimen can be adjusted by changing the water to cement ratio of the concrete to suit the capacity of your test set up. The length of curing can also be varied.

The amount of force needed to produce failure in a beam depends on the beam's dimensions. The theoretical maximum tensile strength of a beam can be calculated by:

The amount of force needed to produce failure in a cylinder depends on the cylinder's cross-sectional area. The theoretical maximum compressive strength of a cylinder can be calculated by:

Some common values needed for failure are:
Beam - 20MN/m² (3000 psi) Cylinder - 35 MN/m² (5000 psi)

The experiment could be used to show the development of strength over time. The students could make several cylinders and beams from each of their concrete batches and the compression strength could be tested over a long period of time such as 1 day, 3 days, 7 days, and 28 days.

The principle of reinforced concrete could be demonstrated by placing a strip of hardware cloth (galvanized steel screen) or chicken wire into the beam in the tension zone.

A cost factor could be incorporated into this lab to make it a true engineering project. The competition could involve assigning points based on most strength for lowest cost.

We offer three testing apparatus designs for measuring the compression strength of the cylinders. Each of these designs is pictured and described on the following pages.

Low Tech Compression Testing Apparatus - The Cement Cracker

Two 8 feet long 2 x 4's were hinged together using a large gate hinge. The hinge plates will need to be bent so as to fit to the 2 x 4 resulting in a device similar to a nut cracker. A separate 2 x 4 which is about two feet long should be nailed to the bottom side of one of the 8' long boards to provide stability. Otherwise, the unit tends to roll to the side. To provide additional strength to the 8' long boards, we attached two small pieces of sheet metal at the location where the cylinders would be seated for testing, about 2 or 3 inches from the hinge. To insure uniform pressure acting on the cylinders, copper pipe caps were placed over the ends of the concrete cylinders. Before placing the copper caps on the cylinders, rubber inserts were placed inside the copper pipe caps to cushion the ends of the cylinders. Place the copper capped concrete cylinders on the sheet metal pads and apply a force on the end of the 8' long boards. By calculating the mechanical advantage of the lever and knowing the applied force, the force exerted on the cylinders can be determined.

Sample calculation: Assuming the cylinder is placed 3" from the hinge on an 8' (96") long board, the mechanical advantage of the lever is 32

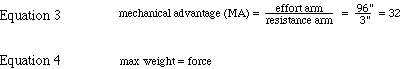
If a 100 pound student stands at the end of the 8' long board, the force exerted on the cylinder is 3200 pounds. If this is attempted, ensure the safety of the student.

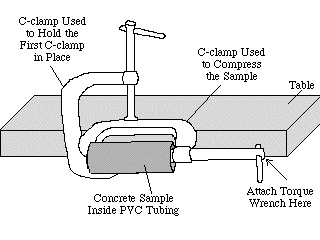
Medium Tech Testing Apparatus - The C-Clamp

Rubber inserts are first placed inside copper pipe caps to provide a cushion for the concrete cylinders. The copper pipe capped cylinder is placed inside a piece of 1" diameter PVC pipe (clear or white) which has been cut long enough to completely cover the concrete cylinder. The PVC pipe is used as a safety means to prevent pieces of concrete from flying outwards when the cylinder breaks. It also provides stability to the test cylinder. Any other piece of pipe that has an inside diameter greater than that of the copper pipe caps could be used. The PVC covered cylinder is placed in the throat of a large (6" or greater) C-clamp. Tighten the threaded shaft onto the copper caps on the cylinder. This entire set up is then clamped to a table top using a second C-clamp. A socket which fits a torque wrench is fitted over the handle of the C-clamp. This socket will need to have slots cut into it so that it snugly fits the handle of the C-clamp. The handle of the C-clamp can be tightened further down onto the concrete cylinder. The torque value can be recorded from the torque wrench. Because the cylinders break rather quickly, we suggest that a video camera is used to record the readings on the torque wrench and played back to obtain more accurate readings. By calculating the mechanical advantage of the C-clamp screw and knowing the torque applied, the force exerted on the cylinder can be determined.

Sample Calculation: The mechanical advantage of a screw can be calculated by:

Video Clip

The mechanical advantage of the screw multiplied by the torque reading equals the maximum force applied to the cylinder, neglecting friction.

High Tech Testing Apparatus - Hydraulic Jack

A hydraulic jack with a pressure gauge provides the easiest method for determining the force exerted on the concrete cylinders. Brodhead-Garrett Co. ( 223 S. Illinois Ave., Mansfield, OH 44901) sells a hydraulic jack with a pressure gauge and platform for about $100. (Dan Phillip 1-800-949-8324)

Place rubber inserts inside copper pipe caps to provide a cushion for the concrete cylinders. Cap the concrete cylinder and place in the platform of the jack. Begin applying pressure while observing the pressure gauge. Allow time between pumps for the cylinders to fail - a minute or two. Safety glasses are a must as pieces of the cylinder fly outward!! A safety shield around the sample would also be a good idea. A video camera may be used to record readings on the pressure gauge and replayed to obtain more accurate readings.

To calculate the compressive strength that is applied to the cylinder, use the following equation.

Video Clip

Expected Results:

For 1/2" cylinders, the cement paste broke at 2000+ psi, gravel at 2000 psi, and vermiculite at 400 psi. The beams' maximum load will vary depending on the form used. Loads as heavy as 100 kilograms have been observed!

Answers to Questions:

1. Top layer, because it is in tension and concrete is week in tension.
2. The top layer will be in compression. The bottom will be in tension.
3. The columns of bridges are concrete while the decking is usually steel. The columns are only under compression while the decking is under tension.
4. a. See equation #1
   b. See equation #2
5. Answers will vary, units will either be psi or N/meter²
6. a. See equations #3 and #4 for low tech apparatus and equation #5 for medium and high tech apparatus.
   b. Answers will vary, units should be newtons or pounds
7. and 8. answers might include: use different aggregate, vary the amounts of components used in the cement, use a different form and change the dimensions.